Scientific journal

International Journal of Experimental Education

ISSN 2618–7159

ИФ РИНЦ = 0,425

Analyzed in the third chapter of the stress state of the columns move to the calculation of stress concentration factors arising from these forces and loads. As it was shown earlier, all current strength and stress in assessing the state of stress of the support elements of the unit it is advisable to lay axes. And these forces caused by normal and shear stresses have to be considered depending on the shape of their action (compression, stretching, bending and torsion).

In the calculations, it should be noted that the elements of the platform are in three areas: water area; splash zone; submarine zone;

Given that, according to [13] the rate of corrosion processes occurring on the columns, is made in accordance with Table 1.

Table 1

The values of corrosion rates, depending on the areas of marine corrosion

We calculate values for determining the values of stress concentration factors for the columns supporting the SME block from the longitudinal compressive or tensile force. Define the following initial values (Table 2.)

Consider a column diameter of 720 mm and a wall thickness in the initial period of operation of 20 mm. We determine the magnitude of the stress concentration factor K based on the fact that the longitudinal compressive force N (down of its own weight, buoyancy aqueous medium, the weight of equipment fouling, etc.) slightly depends on reducing the diameter of the column.

Table 2

The initial values

Viewed element is in the underwater area, the corrosion rate in this case, according to Table 2 0,12 mm/year.

Stress concentration factor is equal to m:

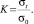(1)

Compressed or stretched nominal stress, according to [10]:

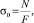(2)

where σ0 – compressed or stretched nominal stress; N – compressive or tensile force; F-sectional area of the pipe.

Given the formula determines the cross sectional area, we get:

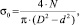(3)

where n D and d – External and inner diameters of the component of the support block and the other values are the same as in (2)

Since the surface corrosion in the offshore field leads to a thinning of the outer surface of the structural elements of SMEs, reducing the value of the outer diameter D, type the value

x = D – 2Vt, mm,

which takes into account the rate of corrosion, and thus obtain the formula to calculate the compressive stress in the period t years:

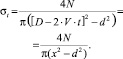(4)

Substituting formula (1) and 2) in (3), we obtain the stress concentration factor for the period of operation of 5 years:

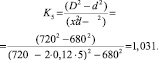(5)

Therefore, we can conclude that the 5-year increase in the nominal stress 1,031 times.

Similarly we calculate the stress concentration factors for ten, fifteen, t wenty, twenty, twenty-five, thirty years and we get the following values: K10 = 1,065; K15 = 1,101; K20 = 1,14; K25 = 1,181; K30 = 1,225. Proceeding similarly, given investigate column located in the variable wetting corrosion rate in this case is 0,16 mm/year (Table 2). The analysis of materials project revealed that the column is usually carried out from the pipes 720h16, 720h20, 720h25, 1020h20, 1020h25 and 1020h30. We calculate stress concentration factors in a longitudinal stressed state and the data are summarized in Table 3.

Analyze the state of stress caused by the bending stress on the wave load (in-depth analysis, see chap. 3). Guided by the above considerations, we derive a formula for estimating the stress concentrations for columns in a corrosive effect under the action of wave loads and the induced bending moment.

Table 3

Stress concentration factor as a function of operating time for the columns of various diameters and wall thickness in a uniform surface corrosion under the action of compressive and tensile forces

Since the surface corrosion in the offshore field leads to a thinning of the outer surface of the structural elements of SMEs, reducing the value of the outer diameter D, type the value

x = D – 2Vt, mm,

which takes into account the rate of corrosion, and thus obtain a formula for the calculation of longitudinal bending stress in the period t years:

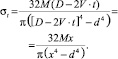(6)

Then the formula for determining the coefficient of stress concentration during the operation period t years will look:

(7)

Let us analyze the shear stresses arising from the action of torque. In the case of wave action on the horizontal elements of the load supporting block ICP, a torque acting on the column.

Nominal shear stresses arising from torsional are:

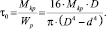(8)

Since the surface corrosion in the offshore field leads to a thinning of the outer surface of the structural elements of SMEs, reducing the value of the outer diameter D, type the value

x = D – 2V∙t, mm,

which takes into account the rate of corrosion, and thus obtain the formula to calculate the shear stresses in the period t years:

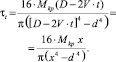(9)

Hence we get the formula for determining the coefficient of stress concentration during the operation period t years:

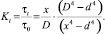(10)

As you can see, the resulting formula describes the concentration of bending stress coincides with the formula describing the stress concentration in torsion. Analysis calculated values KKN showed that their value in cases of the forces of compression, tension and bending or twisting moments are different from each other in the same conditions, no more than 2 %, which allows them to combine in the general formula.

By agreement between the authors copyright and all other rights associated with the defense of a thesis based on this article belong to I.V. Starokon.